Addition Property of Equality: Definition, Properties, and Examples

Understanding the fundamentals of mathematics is necessary for success in the land of numbers since it is the universal language. In this area, the Properties of Equality are crucial since they provide us the ability to work with equations and efficiently solve issues.

We will explore the Addition Properties of Equality in this post. Learn about the foundational ideas behind the Addition Properties of Equality and how they impact mathematics. 

What is the Addition Property of Equality?

The Addition Properties of Equality form the building blocks upon which algebraic equations are constructed. These properties govern the rules of adding or subtracting values from both sides of an equation while maintaining its equality.

Mathematicians may simplify complicated equations, isolate variables, and solve mathematical issues precisely and precisely by comprehending and using these qualities.

Properties of Equality:

The Reflexive Property of Equality:

If you have a number or letter called "x," then "x = x." This might seem obvious, but it's important because it helps us do more math stuff later on.

The Symmetric Property of Equality:

Without affecting an equation's equality, the symmetric property emphasizes the interchangeability of values on both sides. If "a = b," the Symmetric Property argues that "b = a." This characteristic enables us to change the order of terms in an equation without compromising the equation's correctness.

The Transitive Property of Equality:

The continuation of equality among several words is guaranteed by the transitive property. "A = c," according to the Transitive Property, if "a = b" and "b = c." This trait enables us to link together various equations and make inferences about their interrelationships.

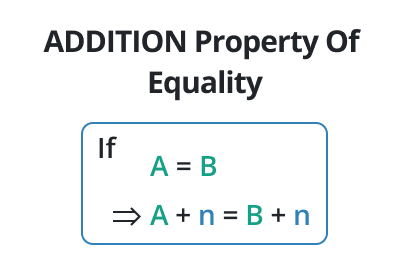
The Addition Property of Equality:

We may add the same amount to both sides of an equation using the Addition Property of Equality without upsetting its equilibrium. If "a = b," we may add the same number "c" to both sides to get "a + c = b + c," which means "a + c = b + c." The foundation of addition-based algebraic operations is this characteristic.

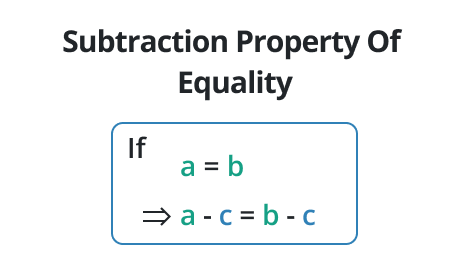
The Subtraction Property of Equality:

The Subtraction Property of Equality allows us to remove the same value from both sides of an equation while maintaining its equality, just like the Addition Property does. If "a = b," we can then take the number "c" out of both sides to get "a - c = b - c." The Addition Property's equivalent is this property.

The Multiplication Property of Equality:

By multiplying both sides of an equation by the same non-zero number, we can keep the equation's balance thanks to the Multiplication Property of Equality. If "a = b," then we can multiply both sides by "c," giving us "ac = bc." This property is particularly useful when solving equations involving multiplication.

The Division Property of Equality:

As the inverse of the Multiplication Property, the Division Property of Equality permits us to divide both sides of an equation by the same non-zero value. If "a = b," then we can divide both sides by "c," resulting in "a/c = b/c." This property complements the Multiplication Property and helps us tackle equations involving division.

FAQs about the Addition Properties of Equality:

Q 1: How do the Addition Properties of Equality affect problem-solving?

   A: We have the skills to accurately reduce equations, isolate variables, and solve mathematical problems thanks to the additional properties of equality. We can break down difficult tasks into small steps by using these features.

Q 2: Can the Addition Properties of Equality be used in real-life situations?

   A: Absolutely! The Addition Properties of Equality are applicable not only in the realm of mathematics but also in various real-life scenarios. From balancing financial transactions to analyzing scientific data, these properties enable us to make accurate calculations and draw meaningful conclusions.

Q 3: Are there any limitations to the Addition Properties of Equality?

   A: While the Addition Properties of Equality are helpful techniques, they operate within certain constraints. For instance, dividing by zero violates these properties and leads to undefined results. Additionally, applying the properties to non-numeric values may yield unexpected outcomes.

Q 4: How can I develop a deeper understanding of the Addition Properties of Equality?

   A: To enhance your understanding, practice applying the properties to a wide range of equations and problems. Work through exercises, seek out additional resources, and engage in discussions with fellow learners or teachers. Building a strong foundation through hands-on practice is key.

Q 5: Can I rely solely on the Addition Properties of Equality to solve all equations?

   A: While the Addition Properties of Equality are powerful problem-solving tools, certain equations may require additional techniques or specialized methods. It is important to develop a diverse toolkit of mathematical strategies to approach different types of problems effectively.

Conclusion

The Addition Properties of Equality serve as the backbone of algebraic manipulations, allowing mathematicians to simplify equations and solve complex problems. By understanding the Reflexive, Symmetric, Transitive, Addition, Subtraction, Multiplication, and Division Properties, you can unlock the potential to master algebra and its applications in various domains.